Connected Funnels

1. There is a motor that keeps the big wheel spinning.

2. There is a connection between small and big : flow / transmission.

3. The big wheel acts like a Flywheel with lots of potential energy.

-- Falcao Soliton / U-Tube --

4. Now for the transmission between the big and the small wheel, like a bike, you need a chain/flow and they have to be able to rotate at dependently. This you get when you have a diagonal flow which is the case for the vortices in the water, when there’s a sinkhole or a connection.

5. So there are two different wheels with two different velocities.

6. To get this you need a structure (which is generated by the movement with the dinner plate).

7. This all brings us to the Torus which is a large rotating Flywheel, and a small inner wheel string-shape : Funnel.

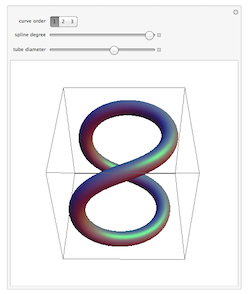
8. Same thing for the fig. 8

9. The string in between also causes for dissipation so everything is in balance and can keep on going. Suction on top with the Open funnels, Dissipation along the wire.

10. The different rotational direction of the 2 U-turn vortices keeps the connection flow in balance, like the chain-link of a bike but twisted.

11. There’s an overal equilibrium state thanks to a 3 Dimensional structure.

Extra Dimensions (V=1)

• 1D: you can keep a steady line; with a velocity in the x=1
• 2D: you can keep a stationary formations: square, circle … or a wavy path; the velocity becomes a combination of xy
• 3D: to move those rotating 2D formations forward you need a 3th dimension (xy-z). Because if you move a rotating formation forward in 2D, every particle starts to have a different velocity, only by adding an extra dimension is it possible to move them together forward in a particular direction, and keep their formation rotation going.
• 4D: to move those round or forward moving formations, one needs again an extra dimension; xyza

Nutcracker

Closed Hilbert Curve

After finding a topic on Continuous Fractal Space-Filling (Hilbert) Curves ...

... in a math-book in the library this week ...

... I started looking for them on the internet and came across a neat tool on the Wolfram-site:

http://demonstrations.wolfram.com/InterpolatingTheHilbertCurveWithABSpline/

(CDF player: http://www.wolfram.com/cdf-player/ - 200 Mb or so)

But because the curve was open, I asked the developer Dr. Michael Trott if he could ad a closing option, like the picture in the book … and hey! he send me this adjusted cdf-file/code where the loop is closed ... et voilà there was the Fig. 8 knot, check it out:
http://800million.org/InterpolatingTheHilbertCurveWithABSplineClosed.cdf